I love the idea of self-reference, words or sentences that refer to themselves in some manner or another. For instance consider the sentence,

This is a sentence.

This is an example of a relatively benign self-referential sentence. Other examples may not be less well-mannered… in fact some sentences can be downright pathological. For instance consider this one:

This sentence contains two erors.

At first blush, it appears that this sentence has only one error – namely the spelling of the word “error” which has been erronously been spelt “erors.” But wait a minute… there is a second error. The fact that it mistakenly claims two errors when actually there is just one! Hmm… so the sentence does have two errors (one of spelling and the other of miscounting the errors).

But… but… but… if it does count the error in counting errors, that means there is just one error in the sentence – which means that the sentence actually mis-counts the number of errors!

Hmmm… I guess we are stuck in a nice little paradox! A paradox that emerges out of self-referential nature of the sentence, a sentence that talks about itself. What fun!

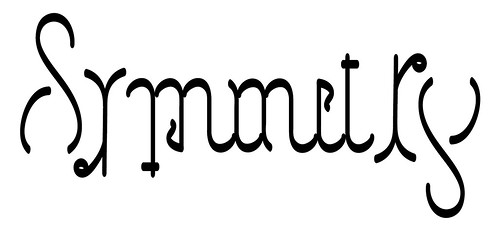
So what does all this have to do with ambigrams? Well ambigrams are usually symmetric – so a nice self-referential ambigram would speak to its symmetric nature in some way. So here it is, an ambigram for the word “symmetric” – which is itself (rotationally) symmetric!